Applet: Line integral of helix mass from density
Applet loading
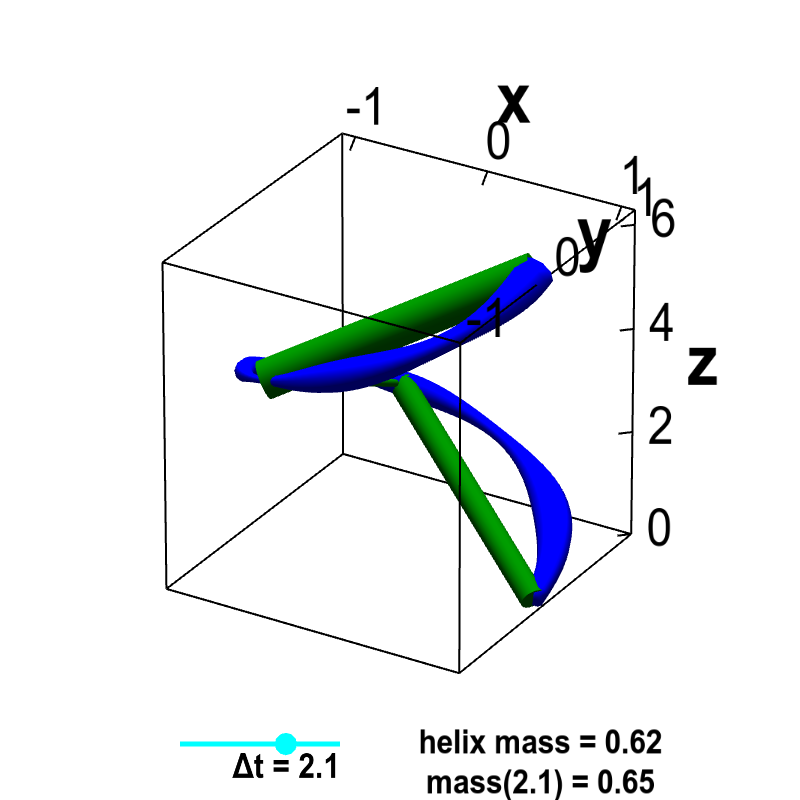
The function $\dllp(t) = (\cos t, \sin t, t)$, for $0 \le t \le 2\pi$ parametrizes a helix. The blue curve representing the helix is drawn with a variable width, representing the varying density $\dlsi(x,y,z)$ of the helix at each point $(x,y,z)=\dllp(t)$ along its length. The green line segments of constant width represent segments of constant density that approximate the helix. The discretization size of the line segments $\Delta t$ can be changed by moving the cyan point on the slider. As $\Delta t \to 0$, the mass of the line segment approximation, labeled mass($\Delta t$), approaches the actual mass of the helix.
Applet links
This applet is found in the pages
Notation systems
More information on notation systems
General information about three.js applets
The applet was made using three.js and requires Javascript as well as a browser that supports WebGL. For most three.js applets, you can drag with the mouse to rotate the view, drag with the right button to pan, and zoom in/out with the mouse wheel. Many applets contain points that you can drag to change values of variables.