Illustration of how the polar coordinate transformation maps a rectangle onto on the Cartesian plane and changes area.
Highlighted pages
- Parametrized curve and derivative as location and velocity
Description of a parametrization of a curve as the position of a particle and the derivative as the particle's velocity. Illustrated with animated graphics. - The idea behind Green's theorem
Introduction to Green's theorem, based on the intuition of microscopic and macroscopic circulation of a vector field. - Parametrization of a line
Introduction to how one can parametrize a line. Interactive graphics illustrate basic concepts. - An introduction to parametrized curves
An introduction to how a vector-valued function of a single variable can be viewed as parametrizing a curve. Interactive graphics illustrate the way in which the function maps an interval onto a curve. - The idea behind Stokes' theorem
Introduction to Stokes' theorem, based on the intuition of microscopic and macroscopic circulation of a vector field and illustrated by interactive graphics.
Recent pages
- A birth-death process
Added April 13, 2022 - A stochastic process introduction
Added April 13, 2022 - An introduction to neural coding and decoding
Added April 3, 2022 - More new items
Highlighted applets
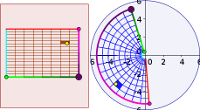
Illustration of how the polar coordinate transformation maps a rectangle onto on the Cartesian plane and changes area.
The dynamics of an undamped pendulum illustrate a two-dimensional state space of a continuous dynamical system.
Welcome to Math Insight
The Math Insight web site is a collection of pages and applets designed to shed light on concepts underlying a few topics in mathematics. The focus is on qualitative description rather than getting all technical details precise. Many of the pages were designed to be read even before students attend lecture on the topic, so they are intended to be somewhat readable introductions to the basic ideas.
You can browse the pages organized into threads, which are sequences through a subset of pages organized by particular topics. An index can help you find pages discussing a particular term. You can also search through the pages, applets, and image captions. A few pages allow you to change the notation system used to render the mathematics.
We hope Math Insight can help you understand key mathematical concepts. We welcome comments on how we can improve it.