Math Insight

Line integrals as circulation

The vector line integral introduction explains how the line integral $\dlint$ of a vector field $\dlvf$ over an oriented curve $\dlc$ “adds up” the component of the vector field that is tangent to the curve. In this sense, the line integral measures how much the vector field is aligned with the curve. If the curve $\dlc$ is a closed curve, then the line integral indicates how much the vector field tends to circulate around the curve $\dlc$. In fact, for an oriented closed curve $\dlc$, we call the line integral the “circulation” of $\dlvf$ around $\dlc$: \begin{align*} \dlint = \text{circulation of $\dlvf$ around $\dlc$}. \end{align*} Sometimes one might write the integral as \begin{align*} \oint_{\dlc} \dlvf \cdot d\lis \end{align*} to emphasize that the integral is around a closed curve, but we tend to omit the circle decoration on the integral sign since it is redundant.

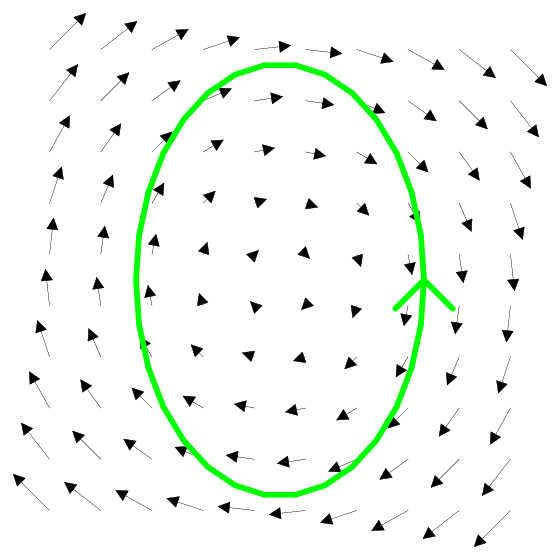
The circulation can be positive or negative, depending on the orientation of $\dlc$ compared to the flow of $\dlvf$. For example, let $\dlvf(x,y) = (y,-x)$ and $\dlc$ be the ellipse \begin{align*} \frac{x^2}{4}+\frac{y^2}{9} =1 \end{align*} oriented counterclockwise. As shown in the graph, the vector field appears to circulate in the clockwise direction, tending to point in the opposite direction of the orientation of the curve. We expect the circulation $\dlint$ to be negative.

We can compute the circulation by parametrizing $\dlc$ by \begin{align*} \dllp(t) = (2\cos t, 3 \sin t) \end{align*} for $0 \le t \le 2\pi$. Since $\dllp'(t) = (-2\sin t, 3 \cos t)$, the line integral is \begin{align*} \dlint &= \plint{0}{2\pi}{\dlvf}{\dllp}\\ &= \int_0^{2\pi} \dlvf(2\cos t, 3 \sin t) \cdot (-2\sin t, 3 \cos t) dt\\ &= \int_0^{2\pi} (3 \sin t, -2\cos t) \cdot (-2\sin t, 3 \cos t) dt\\ &=\int_0^{2\pi} (-6 \sin^2t -6 \cos^2t) dt = \int_0^{2\pi} -6 dt = -12\pi. \end{align*}

In other cases, the circulation may not be so obvious in a picture. For example, let $\dlvf(x,y) = (-y,0)$ and let $\dlc$ be the counterclockwise oriented unit circle, as pictured below.

In this case, there is no obvious circulation of $\dlvf$. However, if you look closely at the alignment of the vector field, you will see that it tends to align with the orientation of the curve. The circulation of $\dlvf$ around $\dlc$ is positive.

We verify this by calculating directly the circulation. Parametrizing the unit circle by $\dllp(t)=(\cos t, \sin t)$ for $0 \le t \le 2\pi$, the circulation is \begin{align*} \dlint &= \plint{0}{2\pi}{\dlvf}{\dllp}\\ &= \int_0^{2\pi} \dlvf(\cos t, \sin t) \cdot (-\sin t, \cos t) dt\\ &= \int_0^{2\pi} (- \sin t, 0) \cdot (-\sin t, \cos t) dt\\ &=\int_0^{2\pi} \sin^2t dt = \int_0^{2\pi} \frac{1-\cos 2t}{2} dt = \pi. \end{align*}