A Möbius strip is not orientable

A surface is orientable if it has two sides. Then, one can orient the surface by choosing one side to be the positive side.

Some unusual surfaces however are not orientable because they have only one side. One classical examples is called the Möbius strip. You can construct a Möbius strip by taking a strip of paper, twisting it half a turn, and then taping the ends together. A Möbius strip with a normal vector is shown below.

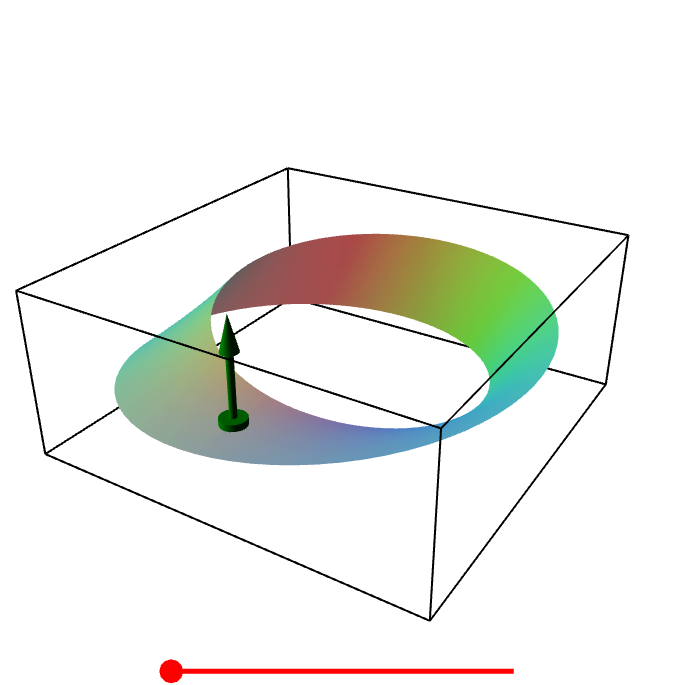
The Java applet did not load, and the above is only a static image representing one view of the applet. The applet was created with LiveGraphics3D. The applet is not loading because it looks like you do not have Java installed. You can click here to get Java.

A Möbius strip is not orientable. A Möbius strip is shown with a normal vector. If you drag the red point on the slider, the normal vector moves along the Möbius strip. Once you drag the slider half way, the normal vector has moved all the way around the surface. However, now the normal vector is pointing in the opposite direction. Since the normal vector didn't switch sides of the surface, you can see that Möbius strip actually has only one side. For this reason, the Möbius strip is not orientable.