Calculation of the surface area of a parametrized surface

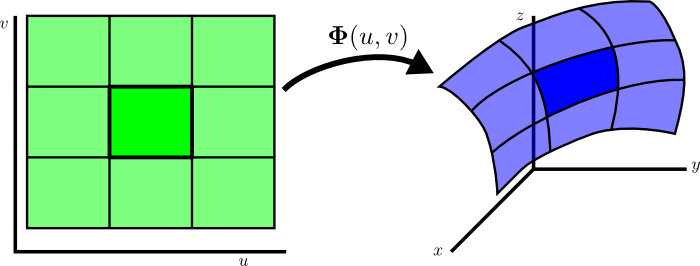
A parametrized surface is a mapping by a function $\dlsp: \R^2 \to \R^3$ (confused?) of a planar region $\dlr$ onto a surface floating in three dimensions. To calculate the area of this surface, we chop up the region $\dlr$ into small rectangles, as displayed below for the function \begin{align*} \dlsp(\spfv,\spsv) = (\spfv\cos \spsv, \spfv\sin \spsv, \spsv). \end{align*}.

The Java applet did not load, and the above is only a static image representing one view of the applet. The applet was created with LiveGraphics3D. The applet is not loading because it looks like you do not have Java installed. You can click here to get Java.

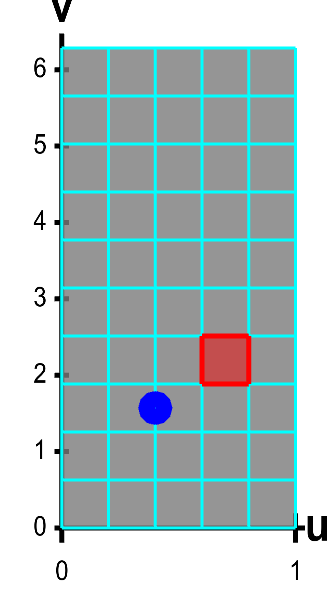
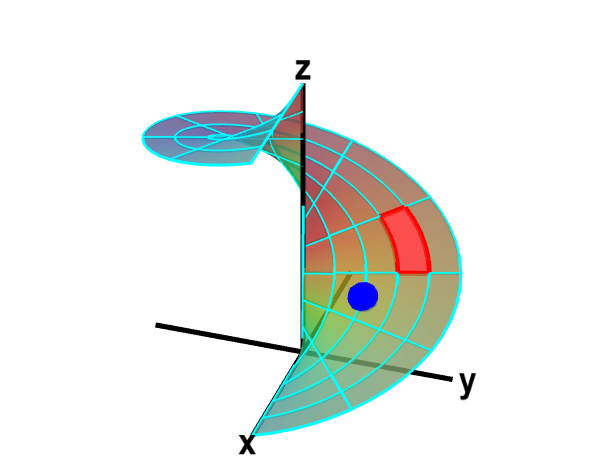
A parametrized helicoid with surface area elements. The function $\dlsp(\spfv,\spsv) = (\spfv\cos \spsv, \spfv\sin \spsv, \spsv)$ parametrizes a helicoid when $(\spfv,\spsv) \in \dlr$, where $\dlr$ is the rectangle $[0,1] \times [0, 2\pi]$. The region $\dlr$ is shown as the green rectangle floating above the helicoid, and it is divided into small rectangles. As you drag the green point in $\dlr$ to specify both $\spfv$ and $\spsv$, a surrounding small rectangle is outlined in green. This rectangle is mapped into the small region of the helicoid that is outlined in red and surrounds the red point $\dlsp(\spfv,\spsv)$. The area of the red region on the helicoid depends on how $\dlsp$ stretches or shrinks the small green rectangle as it maps it on the surface.

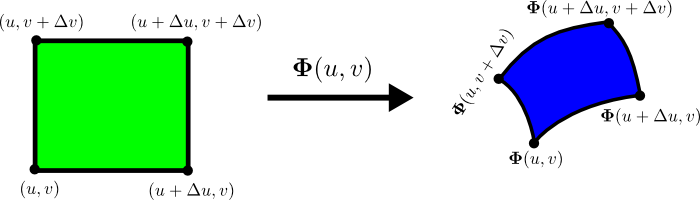
The function $\dlsp$ maps each small rectangle to a “curvy rectangle” on the surface. Here's another view of this mapping, where you have to imagine that the blue surface on the right is floating in space.

Let $\Delta \spfv$ and $\Delta \spsv$ be width and height of the small rectangle. If the lower-left corner of the rectangle is at position $(\spfv,\spsv)$, then $\dlsp$ maps the lower-left corner to the point $\dlsp(\spfv,\spsv)$ on the “curvy rectangle.”

The lower-right corner of the rectangle is the point $(\spfv+\Delta \spfv,\spsv)$, so it gets mapped to $\dlsp(\spfv+\Delta \spfv,\spsv)$. Similarly, the upper-left corner of the rectangle is mapped to $\dlsp(\spfv,\spsv + \Delta \spsv)$.

To calculate the area of the “curvy rectangle”, i.e., the image of the small rectangle under $\dlsp$, we approximate it as a parallelogram. This approximation is okay when $\Delta \spfv$ and $\Delta \spsv$ small.

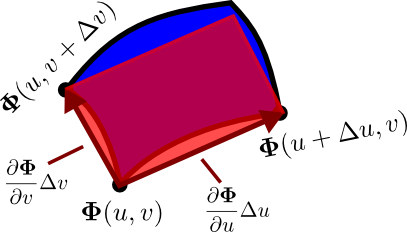
One side of the parallalogram is \begin{align*} \dlsp(\spfv+\Delta \spfv,\spsv)-\dlsp(\spfv,\spsv) &= \frac{\dlsp(\spfv+\Delta \spfv,\spsv)-\dlsp(\spfv,\spsv)}{\Delta \spfv} \Delta \spfv\\ &\approx\pdiff{\dlsp}{\spfv}(\spfv,\spsv)\Delta \spfv \end{align*} when $\Delta \spfv$ is small. Similarly, when $\Delta \spsv$ is small, the other side of the parallelogram is approximatly \begin{align*} \dlsp(\spfv,\spsv+\Delta \spsv) - \dlsp(\spfv,\spsv) \approx \pdiff{\dlsp}{\spsv}(\spfv,\spsv)\Delta \spsv. \end{align*}

The area of a parallegram spanned by these vectors is the magnitude of their cross product. The area of the “curvy rectangle” is approximately \begin{align*} \Delta A=\left\|\pdiff{\dlsp}{\spfv}(\spfv,\spsv) \times \pdiff{\dlsp}{\spsv}(\spfv,\spsv)\right\| \Delta \spfv \Delta \spsv. \end{align*}

The area of the whole surface is a Riemann sum over all the small rectangles. Each term is of the above form. If we take the limits $\Delta \spfv, \Delta \spsv \to 0$, then the Riemann sum converges to a double integral, and we find that the total surface area is \begin{align*} A =\left.\iint_\dlr \left\| \pdiff{\dlsp}{\spfv}(\spfv,\spsv) \times \pdiff{\dlsp}{\spsv}(\spfv,\spsv) \right\|\right. d\spfv\,d\spsv \end{align*} as promised.

Notation systems

More information on notation systems